 |
How would we approach an occurrence related to the fourth dimension? Or the fourth dimension in general? Defining what a 4-dimensional plane is involves studying the properties and configuration of planes in lower dimensions. Because there is no empirical evidence supporting spaces higher than our own - higher dimensions being 'hidden' from us in the way that they are - we must do our best with what is presented to us. What this involves, of course, is rigorous study of lower dimensions. In the process of doing this we assume that everything found true for lower dimensions must also be true for our own. As the means of carrying out this method of study, we relate a lower-dimensional arrangement to our dimension in the same way that the lower-dimensional arrangement relates to its own dimension. It is because of the very fact that lower dimensions exist, you see, that this approach is possible. This system of reasoning - the process of analogy - is the means by which we can "fill in the blanks" where there would otherwise be no answers. The use of analogy is the invaluable "tool" with which we can gain knowledge of the fourth dimension and of the 4-dimensional occurrences related to it - knowledge that we could not obtain from what is presented to us by means of sensory experience.
As was stated above, the process of analogy in essence consists of relating a lower-dimensional arrangement to our dimension in the same way that the lower-dimensional arrangement relates to its own dimension. Given this, take note that in future sections, the manner in which a lower-dimensional arrangement is related to our dimension, may be a manner that requires of us to relate to our dimension in ways that we never have before. Upon such an encounter, the new manner in which we are required to relate to our dimension may seem to break the "rules" that our minds have set down dictating to us what is and isn't "spatially possible". These "rules" are the very result of a life confined to the limitations of our own dimension. Do not be alarmed, then, if you come across a structure or concept in this section or in a future section of this material that seems impossible to believe. Expect, rather, such an encounter, and remind yourself that relating to one's dimension in new ways can in fact be difficult. In summary, then, we should realize that if something doesn't seem to make sense in terms of the rules we know this dimension to operate by, it is probably because what we have come across exists outside the very boundaries of our dimension, and cannot be expressed in terms of concepts we can claim to have experienced.
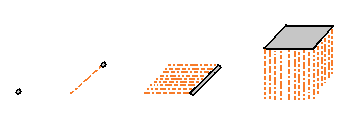
Having been made familiar with the process of analogy and with the ways in which we will relate to it in future sections, we will now go about addressing what is next to come in the presentation of this section. Presented below is the result of an effort to gain the 'knowledge' spoken of earlier in this section - the knowledge that is made possible by the process of analogy. It is done in the spirit of the classic sequence (displayed at the top of this page) that begins with a point, and afterward shows how structures can 'glide' in a direction perpendicular to themselves and leave behind a 'trail' in the process - a trail that in turn becomes the next structure. Focus, then, your attention on the sequence presented below. Though similar in many ways to the 'gliding structure' sequence presented above, there is in the sequence presented below an aspect not found in the 'gliding structure' sequence - an aspect that allows the sequence to take on deeper meaning: this aspect is involvement with intelligent, thinking lower-dimensional creatures.
These sequences differ in terms of the 'final notion' they present. The 'final notion' presented by the 'gliding structure' sequence is a question: the question of how one 'glides' a cube in a direction perpendicular to itself. The final notion presented by the sequence below, however, is that of a solid conclusion, reached fully through what was observed in the lower dimensions. Provided are not questions, but answers. Upon encountering the 'creature' in each example, try to avoid 'debating' whether each creature could theoretically exist in reality - if necessary, they can be thought of more as 'concepts' than as actual 'beings'. Upon defining what a 4-dimensional plane is (the goal of the sequence) we will possess an overall view of the fourth dimension in general.
A zero-dimensional creature can comprehend nothing beyond a point. The creature is on the central point of an array of points, however, surrounded on both sides by points identical to his own. These points extend outward from the creature's point into the first dimension. Because the creature cannot comprehend extension into these directions, we move the points surrounding the creature's point onto the creature's point, forming what we will call a "compressed" array of points. This "compressed" array of points exists as a group of points all occupying the same space and represents a version of the first dimension that the zero-dimensional creature can comprehend: the concept of multiple instances of his own point.
A 1-dimensional creature can comprehend nothing beyond a 1-dimensional plane. The creature is on the central plane of an array of 1-dimensional planes, however, surrounded on both sides by planes identical to his own. These planes extend outward from the creature's plane into the second dimension. Because the creature cannot comprehend extension into these directions, we move the planes surrounding the creature's plane onto the creature's plane, forming what we will call a "compressed" array of 1-dimensional planes. This "compressed" array of 1-dimensional planes exists as a group of 1-dimensional planes all occupying the same space and represents a version of the second dimension that the 1-dimensional creature can comprehend: the concept of multiple instances of his own plane.
A 2-dimensional creature can comprehend nothing beyond a 2-dimensional plane. The creature is on the central plane of an array of 2-dimensional planes, however, surrounded on both sides by planes identical to his own. These planes extend outward from the creature's plane into the third dimension. Because the creature cannot comprehend extension into these directions, we move the planes surrounding the creature's plane onto the creature's plane, forming what we will call a "compressed" array of 2-dimensional planes. This "compressed" array of 2-dimensional planes exists as a group of 2-dimensional planes all occupying the same space and represents a version of the third dimension that the 2-dimensional creature can comprehend: the concept of multiple instances of his own plane.
We, being 3-dimensional creatures, can comprehend nothing beyond a 3-dimensional plane. If our plane were the central plane of an array of 3-dimensional planes identical to our own, into what directions would the surrounding 3-dimensional planes extend? Much like the creatures in the examples above, we cannot comprehend extension into the fourth dimension! What we can grasp, however, is the array of 3-dimensional planes as it exists in its "compressed" state. It exists in this example in the form of our own 3-dimensional plane and consists of what we would consider to be a group of 3-dimensional planes all occupying the same space. It represents a version of the fourth dimension that we can comprehend: the concept of multiple instances of our own 3-dimensional plane. This would represent our basic conception of the fourth dimension. |
What, then, is the purpose and meaning behind "compressing" planes? One by one, the examples in the sequence above introduce a new dimension of extension in the form of an array of planes. Though the planes extend into very real directions, the given subject who is located on the central plane (in the middle of the occurrence of the extension) cannot comprehend these directions of extension. This is the unavoidable result of the subject's life of confinement to his own dimension. The method of "compressing" planes does not attempt to explain these directions to the subject, for the simple reason that the limitations of the subject's visualization capabilites make comprehending the directions impossible. In response to the subject's inability to comprehend these directions, we turn to a concept that the subject can comprehend: the concept of being surrounded by planes identical to his own. Though the subject can't comprehend the location of these planes, he is nonetheless aware that they exist. The subject overcomes his inability to comprehend the directions that the surrounding planes extend into by approaching the situation from a more basic level: because the planes surrounding the subject are identical to his own plane, the subject in a sense successfully conceives of them by simply visualizing the abstract concept of multiple instances of his own plane . He does this by assuming the planes surrounding his own plane to all occupy the space that he perceives his own plane to take up. Surprisingly, this works. The purpose and meaning behind "compressing" planes is that if this process presents to each subject a successful version of each array of planes in all three lower-dimensional examples, why shouldn't the version that this process presents to our own dimension be equally as valid?
And hence we see that even though a given subject is not capable of comprehending how an array of planes of his own dimension extends into the next dimension, what he can in fact grasp is the array of planes as it exists in its "compressed" state: the subject visualizes all of the planes of the array to occupy the space he perceives his own plane to take up. Though not an exact copy of the original array of planes, this version of the array of planes, as you will see, can accomplish just as much. The "compressed" array of 3-dimensional planes (described above as a group of 3-dimensional planes all occupying the same space) is in fact the '4-dimensional plane' mentioned in the title of this section - and is the means by which we will construct the hypersphere. It is in the next section that we cover the details of how this '4-dimensional plane' relates to the slices of the hypersphere spread across it.
| to previous section |
to table of contents |
to next section |
||
| Comments, questions, feedback: [email protected] |