| The Soma Cube Puzzle - 1 |
It is a beautiful freak of nature that the seven simplest irregular combinations of cubes can form a cube again. Variety growing out of unity returns to unity. It is the world's smallest philosophical system.
Piet Hein, discoverer of the Soma Cube.
In the Summer of 1969, Parker Brothers Inc. produced a commercial version of the puzzle known as the Soma Cube. Previously, the puzzle had been popularized by Martin Gardner, the Mathematical Games columnist for Scientific American . The puzzle can be used to help develop skills in visualizing spatial relationships. If you are unfamiliar with this puzzle, read on, for the puzzle can provide hours of fun and exploration! The Soma Cube's discoverer, Piet Hein, a Danish poet and inventor, first envisioned the cube while attending a lecture on quantum physics. He visualized the set of all irregular shapes formed by no more than 4 cubes, each of the same size.
| The Seven Soma Pieces |
|---|
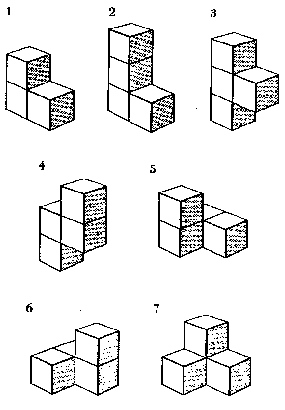
|
The seven possible shapes are shown in the figure at the left. Mr. Hein's insight was that this set of 7 objects could be formed into a larger cube measuring 3 units on a side. Note that if you total the small cubes comprising the 7 Soma pieces, you get 27, the number needed to make a 3x3x3 cube. It is important to note that Mr. Hein did NOT begin with a cube, and cut it up to form the puzzle. He visualized the pieces first, then considered whether they would form a cube.
If the commercial version of the puzzle is not available, a set of Soma pieces can easily be constructed out of wooden cubes, glued at their faces. When you are done, you will have a hands-on manipulative puzzle that will keep any family member entranced and busy for hours (or even days) for there is more to the puzzle than just the cubical structure, as we shall see.
The Basic Soma Puzzle Problem
After obtaining or constructing your seven Soma pieces, you will want to use them to construct the cube itself. As a first step, try to construct this figure using just two of the seven Soma pieces. After you succeed, then its time to try and build the Soma Cube. It can be proven that there are exactly 240 different ways to put the Soma pieces together to form the Soma Cube. The does NOT count rotations or reflections of the cube as being different. That is, you cannot obtain new solutions by simply turning the cube or looking at its mirror image.
One obvious activity is to try to find several possible solutions. It soon becomes clear that a method of recording solutions must be found. One method, which I have used to record solutions, is to look at the completed cube from the top down, and record the number of the piece each component cube belongs to using the piece numbers in the figure above. In recording a solution, three 3x3 squares represent each level of the cube looking down from the top. To illustrate, one possible Soma solution can be found here.. Once you have discovered a solution, record it using the method of the sample.

|
| Castle II |
In addition to the cube, Soma enthusiasts have discovered many figures which can be made from the seven Soma pieces. At the right is one example of a Soma figure. Continue to The Soma Figure Gallery to see other examples. The challenge to the reader is to construct the figures illustrated. Solutions can be represented in a manner similar to that used for the cube solutions, or figures may be drawn. Some solutions for the figures in the Soma Gallery can be found on Page 2. Also, some two set figures are shown on Page 2.
| 1 | Gallery | 2 |